√25 + 10√3
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\left(a\right):2x-7\sqrt{x}+3=0\left(x\ge0\right)\\ < =>\left(2x-6\sqrt{x}\right)-\left(\sqrt{x}-3\right)=0\\ < =>2\sqrt{x}\left(\sqrt{x}-3\right)-\left(\sqrt{x}-3\right)=0\\ < =>\left(2\sqrt{x}-1\right)\left(\sqrt{x}-3\right)=0\\ =>\left[{}\begin{matrix}2\sqrt{x}-1=0\\\sqrt{x}-3=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=\dfrac{1}{4}\left(TM\right)\\x=9\left(TM\right)\end{matrix}\right.\)
\(\left(b\right):3\sqrt{x}+5< 6\\ < =>3\sqrt{x}< 1\\ < =>\sqrt{x}< \dfrac{1}{3}\\ < =>0\le x< \dfrac{1}{9}\)
\(\left(c\right):x-3\sqrt{x}-10< 0\\ < =>\left(x-5\sqrt{x}\right)+\left(2\sqrt{x}-10\right)< 0\\ < =>\sqrt{x}\left(\sqrt{x}-5\right)+2\left(\sqrt{x}-5\right)< 0\\ < =>\left(\sqrt{x}-5\right)\left(\sqrt{x}+2\right)< 0\\ =>\left\{{}\begin{matrix}\sqrt{x}-5< 0\\\sqrt{x}+2>0\end{matrix}\right.\\ < =>\left\{{}\begin{matrix}0\le x< 25\\x\ge0\end{matrix}\right.< =>0\le x< 25\)
\(\left(d\right):x-5\sqrt{x}+6=0\left(x\ge0\right)\\ < =>\left(x-2\sqrt{x}\right)-\left(3\sqrt{x}-6\right)=0\\ < =>\sqrt{x}\left(\sqrt{x}-2\right)-3\left(\sqrt{x}-2\right)=0\\ < =>\left(\sqrt{x}-3\right)\left(\sqrt{x}-2\right)=0\\ =>\left[{}\begin{matrix}\sqrt{x}-3=0\\\sqrt{x}-2=0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x=9\\x=4\end{matrix}\right.\left(TM\right)\)
\(\left(e\right):x+5\sqrt{x}-14< 0\\ < =>\left(x+7\sqrt{x}\right)-\left(2\sqrt{x}+14\right)< 0\\ < =>\sqrt{x}\left(\sqrt{x}+7\right)-2\left(\sqrt{x}+7\right)< 0\\ < =>\left(\sqrt{x}-2\right)\left(\sqrt{x}+7\right)< 0\\ =>\left\{{}\begin{matrix}\sqrt{x}+7>0\\\sqrt{x}-2< 0\end{matrix}\right.\\ < =>\left[{}\begin{matrix}x\ge0\\0\le x< 4\end{matrix}\right.< =>0\le x< 4\)

Đặt \(gcd\left(a,b\right)=d\) và \(lcm\left(a,b\right)=m\) \(\left(d,m\inℕ^∗\right)\). Điều kiện đã cho tương đương \(d+m+a+b=ab\) \(\Leftrightarrow\dfrac{d}{ab}+\dfrac{m}{ab}+\dfrac{1}{a}+\dfrac{1}{b}=1\) (1)
Ta lại có \(dm=ab\) (mình sẽ chứng minh cái này sau) nên từ (1) ta có \(\dfrac{1}{m}+\dfrac{1}{d}+\dfrac{1}{a}+\dfrac{1}{b}=1\) (2).
Do \(d\le b\le a\le m\) nên \(\dfrac{1}{m}\le\dfrac{1}{a}\le\dfrac{1}{b}\le\dfrac{1}{d}\). Kết hợp với (2), ta được \(1=\dfrac{1}{m}+\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{d}\le\dfrac{4}{d}\) \(\Leftrightarrow d\le4\) hay \(d\in\left\{1,2,3,4\right\}\).
Nếu \(d=1\) thì ta có \(\dfrac{1}{m}+\dfrac{1}{a}+\dfrac{1}{b}=0\), vô lí.
Nếu \(d=2\) thì ta có \(\dfrac{1}{m}+\dfrac{1}{a}+\dfrac{1}{b}=\dfrac{1}{2}\), khi đó \(\dfrac{1}{2}=\dfrac{1}{m}+\dfrac{1}{a}+\dfrac{1}{b}\le\dfrac{3}{b}\) nên \(b\le6\) hay \(b\in\left\{1,2,3,4,5;6\right\}\). Dĩ nhiên \(b\) không thể là số lẻ do \(d=2\) là ước của b. Vậy thì \(b\in\left\{2,4,6\right\}\). Nếu \(b=2\) thì \(\dfrac{1}{m}+\dfrac{1}{a}=0\), vô lí. Nếu \(b=4\) thì \(\dfrac{1}{m}+\dfrac{1}{a}=\dfrac{1}{4}\le\dfrac{2}{a}\Leftrightarrow a\le8\) hay \(a\in\left\{1,2,3,4,5,6,7,8\right\}\). Do a cũng là số chẵn nên \(a\in\left\{2,4,6,8\right\}\), mà \(a\ge b\) nên suy ra \(b\in\left\{4,6,8\right\}\). Có \(b=4\) và \(b=6\) thỏa mãn. Nếu \(b=8\) thì \(\dfrac{1}{m}+\dfrac{1}{a}=\dfrac{3}{8}\le\dfrac{2}{a}\Leftrightarrow a\le\dfrac{16}{3}\Leftrightarrow a\le5\), mà \(a\ge b\) nên vô lí
Nếu \(d=3\) thì \(\dfrac{1}{m}+\dfrac{1}{a}+\dfrac{1}{b}=\dfrac{2}{3}\le\dfrac{3}{b}\) \(\Leftrightarrow b\le\dfrac{9}{2}\Leftrightarrow b\le4\) hay \(b\in\left\{1,2,3,4\right\}\). Mà \(b⋮3\) nên \(b=3\). Khi đó \(\dfrac{1}{m}+\dfrac{1}{a}=\dfrac{1}{3}\le\dfrac{2}{a}\Leftrightarrow a\le6\) Nhưng vì \(a⋮3\) nên \(a\in\left\{3,6\right\}\). Nếu \(a=3\) thì thử lại không thỏa mãn. Nếu \(a=6\) thì thỏa mãn.
Nếu \(d=4\) thì \(\dfrac{1}{m}+\dfrac{1}{a}+\dfrac{1}{b}=\dfrac{3}{4}\le\dfrac{3}{b}\) hay \(b\le4\). Mà \(b⋮4\) nên \(b=4\), từ đó suy ra \(\dfrac{1}{m}+\dfrac{1}{a}=\dfrac{1}{2}\le\dfrac{2}{a}\Leftrightarrow a\le4\), vì \(a⋮4\) nên \(a=4\).
Vậy ta tìm được các cặp số (4;4); (4;6); (6;3) thỏa ycbt.
(*) Như mình đã hứa, mình sẽ chứng minh \(gcd\left(a,b\right).lcm\left(a,b\right)=ab\):
Ta biết rằng 1 số tự nhiên N khác 0 bất kì có thể viết được dưới dạng \(N=p_1^{a_1}.p_2^{a_2}...p_n^{a_n}\) với \(p_i\left(i=\overline{1,n}\right)\) là các số nguyên tố đôi một phân biệt còn \(a_i\left(i=\overline{1,n}\right)\) là các số tự nhiên.
Trở lại bài toán, ta đặt \(a=p_1^{m_1}.p_2^{m_2}...p_k^{m_k}\) và \(b=p_1^{n_1}.p_2^{n_2}...p_k^{n_k}\). Khi đó, rõ ràng \(gcd\left(a,b\right)=p_1^{min\left\{m_1,n_1\right\}}.p_2^{min\left\{m_2,n_2\right\}}...p_k^{min\left\{m_k,n_k\right\}}\) và \(lcm\left(a,b\right)=p_1^{max\left\{m_1,n_1\right\}}.p_2^{max\left\{m_2,n_2\right\}}...p_k^{max\left\{m_k,n_k\right\}}\). Do đó \(gcd\left(a,b\right).lcm\left(a,b\right)=\prod\limits^k_{i=1}p_i^{min\left\{m_i,n_i\right\}+max\left\{m_i,n_i\right\}}=\prod\limits^k_{i=1}p_i^{m_i+n_i}=ab\) (kí hiệu \(\prod\limits^k_{i=1}A_i=A_1A_2...A_k\))
, ta có đpcm

P = A.B = \(\dfrac{x-7}{\sqrt{x}+2}=\dfrac{\left(x-4\right)-3}{\sqrt{x}+2}=\dfrac{\left(\sqrt{x}-2\right).\left(\sqrt{x}+2\right)-3}{\sqrt{x}+2}\)
\(=\sqrt{x}-2-\dfrac{3}{\sqrt{x}+2}\)
\(P\inℤ\) <=> x là số chính phương và \(\dfrac{3}{\sqrt{x}+2}\inℤ\)
mà \(\sqrt{x}+2\ge2\Rightarrow\dfrac{3}{\sqrt{x}+2}\inℤ\Leftrightarrow\sqrt{x}+2=3\)
\(\Leftrightarrow x=1\) (thỏa)
Vậy x = 1 thì P \(\inℤ\)

2\(x\) + 5\(\sqrt{x}\) = 3 ( Đkxđ \(x\) ≥ 0)
2\(x\) + 5\(\sqrt{x}\) = 3
⇒2\(x\) + 5\(\sqrt{x}\) - 3 = 0
Đặt \(\sqrt{x}\) = y ( y > 0)
Ta có: 2\(x\) + 5\(\sqrt{x}\) - 3 = 0
⇔2y2 + 5y - 3 = 0
△ = 25 + 24 = 49 > 0
y1 = ( -5 + \(\sqrt{49}\)) : 4 = \(\dfrac{1}{2}\)
y2 = (-5 - \(\sqrt{49}\)): 4 = - 3 (loại)
\(\Rightarrow\) \(\sqrt{x}\) = \(\dfrac{1}{2}\) ⇒ \(x\) = \(\dfrac{1}{4}\)
Vậy \(x\) = \(\dfrac{1}{4}\)

\(\sqrt{x^2-x+2}=x\left(1\right)\)
Ta thấy : \(x^2-x+2>0\) nên không cần ĐKXĐ.
\(\left(1\right)\Leftrightarrow x^2-x+2=x^2\left(ĐK:x\ge0\right)\)
\(\Leftrightarrow x^2-x+2-x^2=0\)
\(\Leftrightarrow-x+2=0\)
\(\Leftrightarrow-x=-2\)
\(\Leftrightarrow x=2\left(tm\right)\)
Vậy pt có tập nghiệm : \(S=\left\{2\right\}\)

Sửa đề : \(\dfrac{a^2}{a^2+b}+\dfrac{b^2}{b^2+a}\le1\\ \) (*)
\(< =>\dfrac{a^2\left(b^2+a\right)+b^2\left(a^2+b\right)}{\left(a^2+b\right)\left(b^2+a\right)}\le1\\ < =>a^2b^2+a^3+b^2a^2+b^3\le\left(a^2+b\right)\left(b^2+a\right)\) ( Nhân cả 2 vế cho `(a^{2}+b)(b^{2}+a)>0` )
\(< =>a^3+b^3+2a^2b^2\le a^2b^2+b^3+a^3+ab\\ < =>a^2b^2\le ab\\ < =>ab\le1\) ( Chia 2 vế cho `ab>0` )
Do a,b >0
Nên áp dụng BDT Cô Si :
\(2\ge a+b\ge2\sqrt{ab}< =>\sqrt{ab}\le1\\ < =>ab\le1\)
Do đó (*) luôn đúng
Vậy ta chứng minh đc bài toán
Dấu "=" xảy ra khi : \(a=b>0,a+b=2< =>a=b=1\)
a Sửa đề : Chứng minh \(\dfrac{a^2}{a^2+b}\)+\(\dfrac{b^2}{b^2+a}\)\(\le\) 1 ( Đề thi vào 10 Hà Nội).
Bất đẳng thức trên tương đương :
\(\dfrac{a^2+b-b}{a^2+b}\)+\(\dfrac{b^2+a-a}{b^2+a}\)\(\le\)1
\(\Leftrightarrow\) 1 - \(\dfrac{b}{a^2+b}\)+ 1 - \(\dfrac{a}{b^2+a}\)\(\le\)1
\(\Leftrightarrow\)1 - \(\dfrac{b}{a^2+b}\) - \(\dfrac{a}{b^2+a}\)\(\le\)0
\(\Leftrightarrow\)- \(\dfrac{b}{a^2+b}\)- \(\dfrac{a}{b^2+a}\)\(\le\)-1
\(\Leftrightarrow\)\(\dfrac{a}{b^2+a}\)+ \(\dfrac{b}{a^2+b}\)\(\ge\)1
Xét VT = \(\dfrac{a^2}{ab^2+a^2}\)+ \(\dfrac{b^2}{a^2b+b^2}\)\(\ge\)\(\dfrac{\left(a+b\right)^2}{ab^2+a^2+a^2b+b^2}\) (Cauchy - Schwarz)
= \(\dfrac{\left(a+b\right)^2}{ab\left(b+a\right)+a^2+b^2}\)
\(\ge\)\(\dfrac{\left(a+b\right)^2}{2ab+a^2+b^2}\)
= \(\dfrac{\left(a+b\right)^2}{\left(a+b\right)^2}\)= 1
Vậy BĐT được chứng minh
Dấu '=' xảy ra \(\Leftrightarrow\)a = b = 1

đkxđ: \(\left\{{}\begin{matrix}x\ne0\\y\ne0\end{matrix}\right.\)
pt đầu \(\Leftrightarrow x+\dfrac{2}{x}+y+\dfrac{1}{y}=6\) (3)
pt thứ 2 \(\Leftrightarrow x^2+\dfrac{4}{x^2}+y^2+\dfrac{1}{y^2}=14\) \(\Leftrightarrow\left(x^2+2.x.\dfrac{2}{x}+\dfrac{4}{x^2}\right)+\left(y^2+2y.\dfrac{1}{y}+\dfrac{1}{y^2}\right)=20\)
\(\Leftrightarrow\left(x+\dfrac{2}{x}\right)^2+\left(y+\dfrac{1}{y}\right)^2=20\) (4)
Đặt \(\left\{{}\begin{matrix}x+\dfrac{2}{x}=u\left(\left|u\right|\ge2\sqrt{2}\right)\\y+\dfrac{1}{y}=v\left(\left|v\right|\ge2\right)\end{matrix}\right.\) thì từ (3) và (4) suy ra \(\left\{{}\begin{matrix}u+v=6\\u^2+v^2=20\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}v=6-u\\u^2+\left(6-u\right)^2=20\end{matrix}\right.\)
\(u^2+\left(6-u\right)^2=20\) \(\Leftrightarrow u^2+36-12u+u^2=20\) \(\Leftrightarrow2u^2-12u+16=0\) \(\Leftrightarrow u^2-6u+8=0\) \(\Leftrightarrow\left(u-2\right)\left(u-4\right)=0\) \(\Leftrightarrow\left[{}\begin{matrix}u=2\left(loại\right)\\u=4\left(nhận\right)\end{matrix}\right.\).
\(\Rightarrow v=6-u=2\), suy ra \(\left\{{}\begin{matrix}x+\dfrac{2}{x}=4\\y+\dfrac{1}{y}=2\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x=2\pm\sqrt{2}\\y=1\end{matrix}\right.\) (nhận).
Vậy hpt đã cho có các nghiệm \(\left(x;y\right)\in\left\{\left(2-\sqrt{2};1\right);\left(2+\sqrt{2};1\right)\right\}\)

\(A=\dfrac{\cos^217^o+2\cos^273^o}{\cot65^o\cot25^o}-\sin^217^o\)
\(A=\dfrac{\left(\cos^217^o+\cos^273^o\right)+\cos^273^o}{\tan25^o\cot25^o}-\sin^217^o\)
(áp dụng công thức \(\cot\alpha=\tan\left(90^o-\alpha\right)\))
\(A=\left(\cos^217^o+\sin^217^o\right)+\sin^217^o-\sin^217^o\)
(áp dụng công thức \(\tan\alpha.\cot\alpha=1\) và \(\cos\alpha=\sin\left(90^o-\alpha\right)\))
\(A=1\)

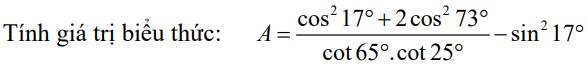
`@` `\text {Ans}`
`\downarrow`
`\sqrt {25} + 10 \sqrt {3}`
`= 5 + 10 \sqrt {3}`
\(\sqrt{25}+10\sqrt{3}=5+10\sqrt{3}\)