Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(a^2+2b^2+3=\left(a^2+b^2\right)+\left(b^2+1\right)+2\ge2ab+2b+2=2\left(ab+b+1\right)\)
Tương tự ...
\(\Rightarrow P\le\dfrac{1}{2\left(ab+b+1\right)}+\dfrac{1}{2\left(bc+c+1\right)}+\dfrac{1}{2\left(ca+a+1\right)}\)
\(=\dfrac{1}{2}\left(\dfrac{c}{abc+bc+c}+\dfrac{1}{bc+c+1}+\dfrac{bc}{ca.bc+a.bc+bc}\right)\)
\(=\dfrac{1}{2}\left(\dfrac{c}{1+bc+c}+\dfrac{1}{bc+c+1}+\dfrac{bc}{c+1+bc}\right)\)
\(=\dfrac{1}{2}\left(\dfrac{c+1+bc}{1+bc+c}\right)=\dfrac{1}{2}\)
\(P_{max}=\dfrac{1}{2}\) khi \(a=b=c=1\)

\(abc=1\) nên tồn tại các số dương x;y;z sao cho \(\left(a;b;c\right)=\left(\dfrac{x}{y};\dfrac{y}{z};\dfrac{z}{x}\right)\)
BĐT cần chứng minh tương đương:
\(\dfrac{y}{x+2y}+\dfrac{z}{y+2z}+\dfrac{x}{z+2x}\le1\)
\(\Leftrightarrow\dfrac{2y}{x+2y}-1+\dfrac{2z}{y+2z}-1+\dfrac{2x}{z+2x}-1\le2-3\)
\(\Leftrightarrow\dfrac{x}{x+2y}+\dfrac{y}{y+2z}+\dfrac{z}{z+2x}\ge1\)
Điều này đúng do:
\(VT=\dfrac{x^2}{x^2+2xy}+\dfrac{y^2}{y^2+2yz}+\dfrac{z^2}{z^2+2xz}\ge\dfrac{\left(x+y+z\right)^2}{x^2+y^2+z^2+2xy+2yz+2zx}=1\)

Đặt \(\left(a;b;c\right)=\left(\dfrac{y}{x};\dfrac{z}{y};\dfrac{x}{z}\right)\)
\(\Rightarrow VT=\dfrac{1}{\dfrac{y}{x}\left(\dfrac{z}{y}+1\right)}+\dfrac{1}{\dfrac{z}{y}\left(\dfrac{x}{z}+1\right)}+\dfrac{1}{\dfrac{x}{z}\left(\dfrac{y}{x}+1\right)}\)
\(VT=\dfrac{x}{y+z}+\dfrac{y}{z+x}+\dfrac{z}{x+y}=\dfrac{x^2}{xy+xz}+\dfrac{y^2}{xy+yz}+\dfrac{z^2}{xz+yz}\)
\(VT\ge\dfrac{\left(x+y+z\right)^2}{2\left(xy+yz+zx\right)}\ge\dfrac{3\left(xy+yz+zx\right)}{2\left(xy+yz+zx\right)}=\dfrac{3}{2}\)

\(\dfrac{1}{\left(a+b+a+c\right)^2}\le\dfrac{1}{4\left(a+b\right)\left(a+c\right)}=\dfrac{1}{4\left(a^2+ab+bc+ca\right)}\le\dfrac{1}{64}\left(\dfrac{1}{a^2}+\dfrac{1}{ab}+\dfrac{1}{bc}+\dfrac{1}{ca}\right)\)
\(\le\dfrac{1}{64}\left(\dfrac{1}{a^2}+\dfrac{1}{a^2}+\dfrac{1}{b^2}+\dfrac{1}{c^2}\right)=\dfrac{1}{64}\left(\dfrac{2}{a^2}+\dfrac{1}{b^2}+\dfrac{1}{c^2}\right)\)
Tương tự và cộng lại:
\(P\le\dfrac{1}{64}\left(\dfrac{4}{a^2}+\dfrac{4}{b^2}+\dfrac{4}{c^2}\right)=\dfrac{1}{16}.3=\dfrac{3}{16}\)
Dấu "=" xảy ra khi \(a=b=c=1\)
Áp dụng bđt: \(\dfrac{1}{x+y}\le\dfrac{1}{4}\left(\dfrac{1}{x}+\dfrac{1}{y}\right)\left(1\right)\)
\(\dfrac{1}{2a+b+c}=\dfrac{1}{\left(a+b\right)+\left(a+c\right)}\le\dfrac{1}{4}\left(\dfrac{1}{a+b}+\dfrac{1}{a+c}\right)\)
\(\Rightarrow P\le\dfrac{1}{16}\left[\left(\dfrac{1}{a+b}+\dfrac{1}{a+c}\right)^2+\left(\dfrac{1}{a+b}+\dfrac{1}{b+c}\right)^2+\left(\dfrac{1}{b+c}+\dfrac{1}{a+c}\right)^2\right]\)\(\Rightarrow16P\le\dfrac{2}{\left(a+b\right)^2}+\dfrac{2}{\left(b+c\right)^2}+\dfrac{2}{\left(a+c\right)^2}+\dfrac{2}{\left(a+b\right)\left(b+c\right)}+\dfrac{2}{\left(a+b\right)\left(b+c\right)}+\dfrac{2}{\left(b+c\right)\left(c+a\right)}\)
Áp dụng: \(x^2+y^2+z^2\ge xy+yz+xz\left(2\right)\) với a+b=x,b+c=y,c+a=z
\(\Rightarrow16P\le\dfrac{4}{\left(a+b\right)^2}+\dfrac{4}{\left(b+c\right)^2}+\dfrac{4}{\left(c+a\right)^2}\)
Ta có: \(\dfrac{1}{\left(a+b\right)^2}\le4.16.\left(\dfrac{1}{a}+\dfrac{1}{b}\right)^2\)(do (1))
\(\Rightarrow16P\le\dfrac{1}{4}.16\left[\left(\dfrac{1}{a}+\dfrac{1}{b}\right)^2+\left(\dfrac{1}{b}+\dfrac{1}{c}\right)^2+\left(\dfrac{1}{c}+\dfrac{1}{a}\right)^2\right]=\dfrac{1}{4}\left(\dfrac{1}{a^2}+\dfrac{1}{b^2}+\dfrac{1}{c^2}+\dfrac{2}{ab}+\dfrac{2}{bc}+\dfrac{2}{ca}\right)\le\dfrac{1}{4}.4.\left(\dfrac{1}{a^2}+\dfrac{1}{b^2}+\dfrac{1}{c^2}\right)=3\)(do(2) và \(\dfrac{1}{a^2}+\dfrac{1}{b^2}+\dfrac{1}{c^2}=3\))
\(\Rightarrow P\le\dfrac{3}{16}\)
\(ĐTXR\Leftrightarrow a=b=c=1\)

TK: Cho các số thực dương a, b, c thỏa mãn a + b+ c = 3. Chứng minh rằng: \(\sqrt{2a^2+\frac{7}{b^2}}+\sqrt{2b^2+\frac{7}{... - Hoc24

\(\dfrac{\sqrt{b^2+a^2+a^2}}{ab}\ge\dfrac{\sqrt{\dfrac{1}{3}\left(b+a+a\right)^2}}{ab}=\dfrac{1}{\sqrt{3}}\left(\dfrac{1}{a}+\dfrac{2}{b}\right)\)
Tương tự: \(\dfrac{\sqrt{c^2+2b^2}}{bc}\ge\dfrac{1}{\sqrt{3}}\left(\dfrac{1}{b}+\dfrac{2}{c}\right)\) ; \(\dfrac{\sqrt{a^2+2c^2}}{ac}\ge\dfrac{1}{\sqrt{3}}\left(\dfrac{1}{c}+\dfrac{2}{a}\right)\)
Cộng vế với vế:
\(VT\ge\dfrac{1}{\sqrt{3}}\left(\dfrac{3}{a}+\dfrac{3}{b}+\dfrac{3}{c}\right)=\sqrt{3}\left(\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{c}\right)=1980\sqrt{3}\)
Dấu "=" xảy ra khi \(a=b=c=\dfrac{3}{1980}\)

b, \(\frac{a^3}{b+2c}+\frac{b^3}{c+2a}+\frac{c^3}{a+2b}\ge1\)
\(\frac{a^4}{ab+2ac}+\frac{b^4}{bc+2ab}+\frac{c^4}{ac+2bc}\ge\frac{\left(a^2+b^2+c^2\right)^2}{ab+bc+ac+2ac+2ab+2bc}\)( Bunhia dạng phân thức )
mà \(a^2+b^2+c^2\ge ab+bc+ac\)
\(=\frac{\left(ab+bc+ac\right)^2}{3+2\left(ab+ac+bc\right)}=\frac{9}{3+6}=1\)( đpcm )
1.
Điều kiện .
Phương trình tương đương với \\
Với ta có:
.
Suy ra .
Vậy phương trình có nghiệm duy nhất
2.
Đặt
Áp dụng bất đẳng thức Cauchy cho hai số dương và ta có
.
Tương tự , .
Cộng các vế ta có .
Mà nên (ta có đpcm).


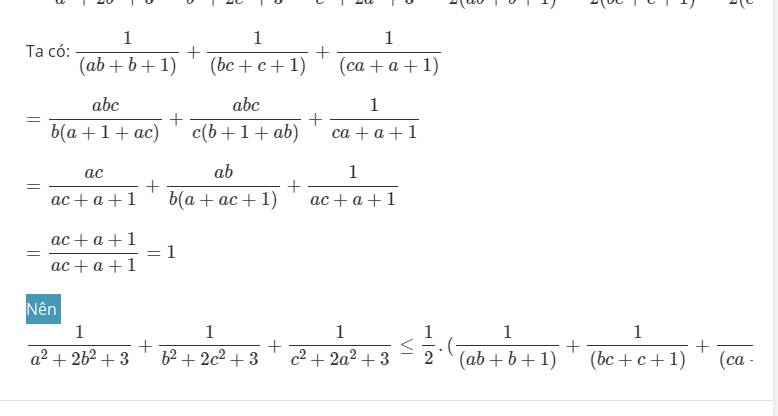

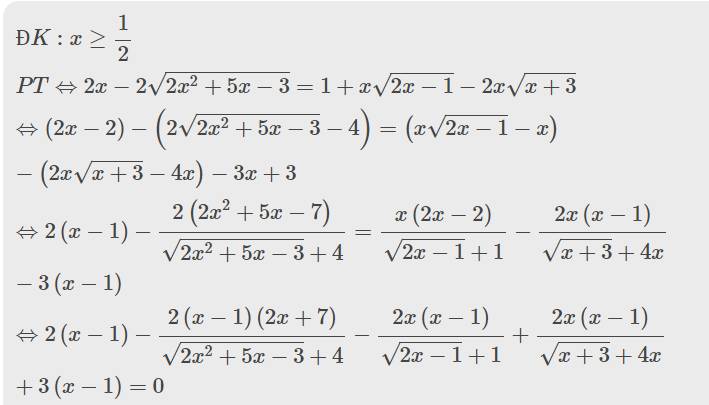


\(Áp\ dụng\ BĐT\ AM - GM,\ ta\ có: \\\sum\dfrac{1}{a^2+2b^2+3}=\sum\dfrac{1}{(a^2+b^2)+(b^2+1)+2}\le\sum\dfrac{1}{2ab+2b+2} \\=\dfrac{1}{2}\sum\dfrac{1}{ab+b+1}=\dfrac{1}{2}.1=\dfrac{1}{2} \\Đẳng\ thức\ xảy\ ra\ khi\ a=b=c=1.\)