Cho tam giác ABC. Trên tia đối của tia AB lấy điểm D sao cho AD = AB, trên tia đối của tia AC lấy điểm E sao cho AE = AC. Gọi M là điểm nằm giữa B và C. Tia MA cắt DE tại N. Chứng minh AM = AN.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Tìm x
(4x - 3) - (x + 5) = 3 (10 - x)
4x - 3 - x - 5 = 30 - 3x
(4x - x) - (3 + 5) = 30 - 3x
3x - 8 = 30 - 3x
3x + 3x = 30 + 8
6x = 38
x = 38 : 6
x = \(\frac{19}{3}\)
Vậy x = \(\frac{19}{3}\).
(4x−3)−(x+5)=3(10−x)
⇒4x−3−x−5=30−3x
⇒3x−8=30−3x
⇒6x=38
⇒x=19/3
Vậy x=19/3
(._.)

em mà hok lớp 7 là em giải ngay nhưng rất tiếc em mới lớp 5
a, f(x) = 9 + 4x - 2x3 + x2 + 7x4
= 7x4 - 2x3 + x2 + 4x + 9
g(x) = 2x2 - 7x4 + 2x3 - 3x - 9
= -7x4 + 2x3 + 2x2 - 3x - 9
b, H(x) = f(x) + g(x)
= 7x4 - 2x3 + x2 + 4x + 9 - 7x4 + 2x3 + 2x2 - 3x - 9
= (7x4 - 7x4) + (2x3 - 2x3) + (x2 + 2x2) + (4x - 3x) + (9 - 9)
= 3x2 + x
P(x) = f(x) - g(x)
= 7x4 - 2x3 + x2 + 4x + 9 - (-7x4 + 2x3 + 2x2 - 3x - 9)
= 7x4 - 2x3 + x2 + 4x + 9 + 7x4 - 2x3 - 2x2 + 3x + 9
= (7x4 + 7x4) - (2x3 + 2x3) + (x2 - 2x2) + (4x + 3x) + (9 + 9)
= 14x4 - 4x3 - x2 + 7x + 18
c, Cho H(x) = 0
\(\Rightarrow\) 3x2 + x = 0
\(\Rightarrow\) x (3x + 1) = 0
\(\Rightarrow\orbr{\begin{cases}x=0\\3x+1=0\end{cases}}\Rightarrow\orbr{\begin{cases}x=0\\x=-\frac{1}{3}\end{cases}}\)

Gọi diện tích miếng đất là \(S\left(m^2\right)\)
Khi đó \(h_a=\frac{2S}{3},h_b=\frac{2S}{4},h_c=\frac{2S}{6}\)
\(h_a-h_b+h_c=25\)
\(\Rightarrow\frac{2S}{3}-\frac{2S}{4}+\frac{2S}{6}=25\)
\(\Leftrightarrow S=25\div\left(\frac{2}{3}-\frac{2}{4}+\frac{2}{6}\right)=50\left(m^2\right)\)

o
o
o
o
o
o
o
o
oo
o
o
o
o
oo
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
oo
o
o
o
o
o
oo
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
oo
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
oo
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
oo
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
ooo
o
o
o
o
o
o
ooo
o
o
o
o
o
oo
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
oo
o
o
o
ooo
o
o
o
o
o
ooo
o
o
oo
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
o
:((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((((=

\(29a+5=31b+28\)
\(\Leftrightarrow29\left(a-b\right)=2b+23\)
\(\Rightarrow2b+23⋮29\)
mà \(x\)nhỏ nhất nên \(b\)nhỏ nhất nên \(2b+23=29\Leftrightarrow b=3\).
Với \(b=3\Rightarrow x=121\).
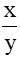
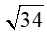 cm. D. 8cm.
cm. D. 8cm.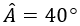 khi đó số đo của góc B bằng
khi đó số đo của góc B bằng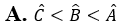
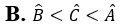
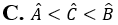
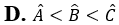

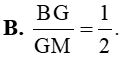
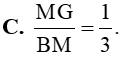
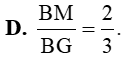
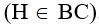 . Khẳng định nào sau đây là đúng?
. Khẳng định nào sau đây là đúng? và
và 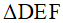 có
có 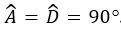 . Để kết luận
. Để kết luận 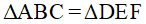 theo trường hợp cạnh huyền – cạnh góc vuông, cần có thêm điều kiện nào sau đây?
theo trường hợp cạnh huyền – cạnh góc vuông, cần có thêm điều kiện nào sau đây?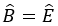
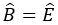
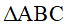 vuông tại A (AB < AC), tia phân giác của góc B cắt AC tại M. Trên tia đối của tia MB lấy điểm D sao cho MB = MD, từ điểm D vẽ đường thẳng vuông góc với AC tại N và cắt BC tại điểm E.
vuông tại A (AB < AC), tia phân giác của góc B cắt AC tại M. Trên tia đối của tia MB lấy điểm D sao cho MB = MD, từ điểm D vẽ đường thẳng vuông góc với AC tại N và cắt BC tại điểm E.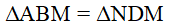 .
.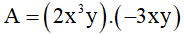
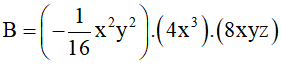
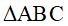 vuông tại A, có AB = 6cm, AC = 8cm.
vuông tại A, có AB = 6cm, AC = 8cm.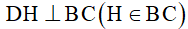 .
.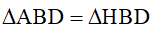
A B C D E M N
Xét \(\Delta ABC\) và \(\Delta ADE\) có:
AB = AD (gt)
\(\widehat{DAE}=\widehat{BAC}\) (2 góc đối đỉnh)
AC = AE (gt)
\(\Rightarrow\Delta ABC=\Delta ADE\left(c.g.c\right)\)
\(\Rightarrow\widehat{B}=\widehat{D}\) (2 góc tương ứng)
Xét \(\Delta ABM\) và \(\Delta ADN\) có:
\(\widehat{B}=\widehat{D}\) (cmt)
AB = AD (gt)
\(\widehat{BAM}=\widehat{DAN}\) (2 góc đối đỉnh)
\(\Rightarrow\Delta ABM=\Delta ADN\left(g.c.g\right)\)
\(\Rightarrow AM=AN\) (2 cạnh tương ứng) (đpcm)